Discontinuous Spectral Element Method (DSEM)

DSEM approximates the solution of the conserved variables on a high-order local basis function inside non-overlapping elements that may be oriented arbitrarily within an unstructured grid. Such discretization makes the method capable of handling complex geometries, local grid refinement, efficient parallelization, and easy boundary condition implementation. The method has been extensively tested for direct numerical simulation (DNS) and LES in our group. DSEM has negligible diffusion and dispersion errors and is spectrally convergent for smooth solutions. The order of approximation, and thus the overall grid resolution, in DSEM can easily be adjusted by simply changing the approximation polynomial order (up to 20) as a single input of the code, without generating a new grid.

DSEM provides an efficient means for stabilizing the simulations, similarly to an LES subgrid model, by explicitly filtering higher modes on the subgrid level. The spectral filtering is performed with maximum computational efficiency and negligible overhead. Explicit filtering methodology is entirely independent of the geometry and is conducted per spectral element without requiring information from neighboring elements. As such, explicit filtering does not impose any overhead on parallelization, and its implementation in complex geometries is straightforward.

Turbulent simulations with DSEM can be conducted using Implicit LES (ILES) via the explicit filtering. Alternately, standard LES models can also be used in DSEM.

Overview

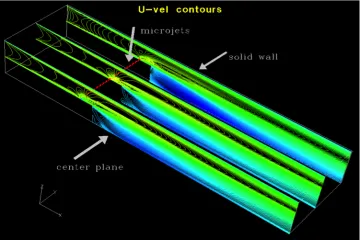
Here you can see an overview of the simplified AFRL Ramp Cavity geometry as simulated by DSEM.

Fourier Spectral Code

Our in-house Fourier spectral code has been used extensively to study turbulent flows laden with a dispersed phase of solid particles or evaporating/reacting droplets. The method relies on periodic boundary conditions that naturally exist in isotropic box turbulence. We have extended the application of Fourier spectral method to homogenous two-phase flows with a constant mean velocity gradient by performing coordinate transformations for both phases. We have studied homogeneous shear flow as well as various axisymmetric flows.

Commercial and Open Source Codes

In addition to our in-house numerical codes, we also use publicly available computational fluid dynamics and heat transfer software prior to simulation using our more accurate research grade codes. This allows for rapid design iteration before investing computational resources.

We have licenses for ANSYS products, including their CFD software Fluent. In addition we have experience using and modifying OpenFOAM, which is an open source finite volume solver, and NEK5000, which is a Spectral Element Method similar to our DSEM.

Image

Modeling

Large Eddy Simulation (LES)

Although our in-house codes are able to perform direct numerical simulation, we typically employ LES models to preserve accuracy of the solution yet save computational time.

Our DSEM code employs a variety of LES models, such as eddy viscosity models, deconvolution models, and an implicit model. The eddy viscosity models rely on the addition of a 'turbulent viscosity' calculated by either the classical Smagorinsky model, dynamic Smagorinsky model, or Modified Kinetic Energy Viscosity (MKEV) model. Due to the ease and low cost of filtering in DSEM, we favor filter based models, such as the scale similarity model and implicit LES.

Overview

The video depicts the mixing of a scalar jet in a backward facing step. The spectral element code employed an LES model, and allowed for local refinement (see near the injector) of the complex geometry.

Probability Density Function (PDF) Modeling

Image

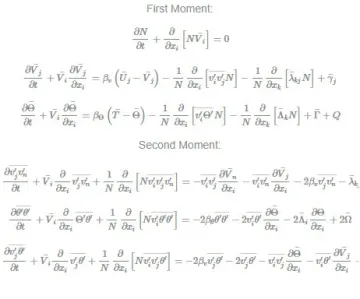
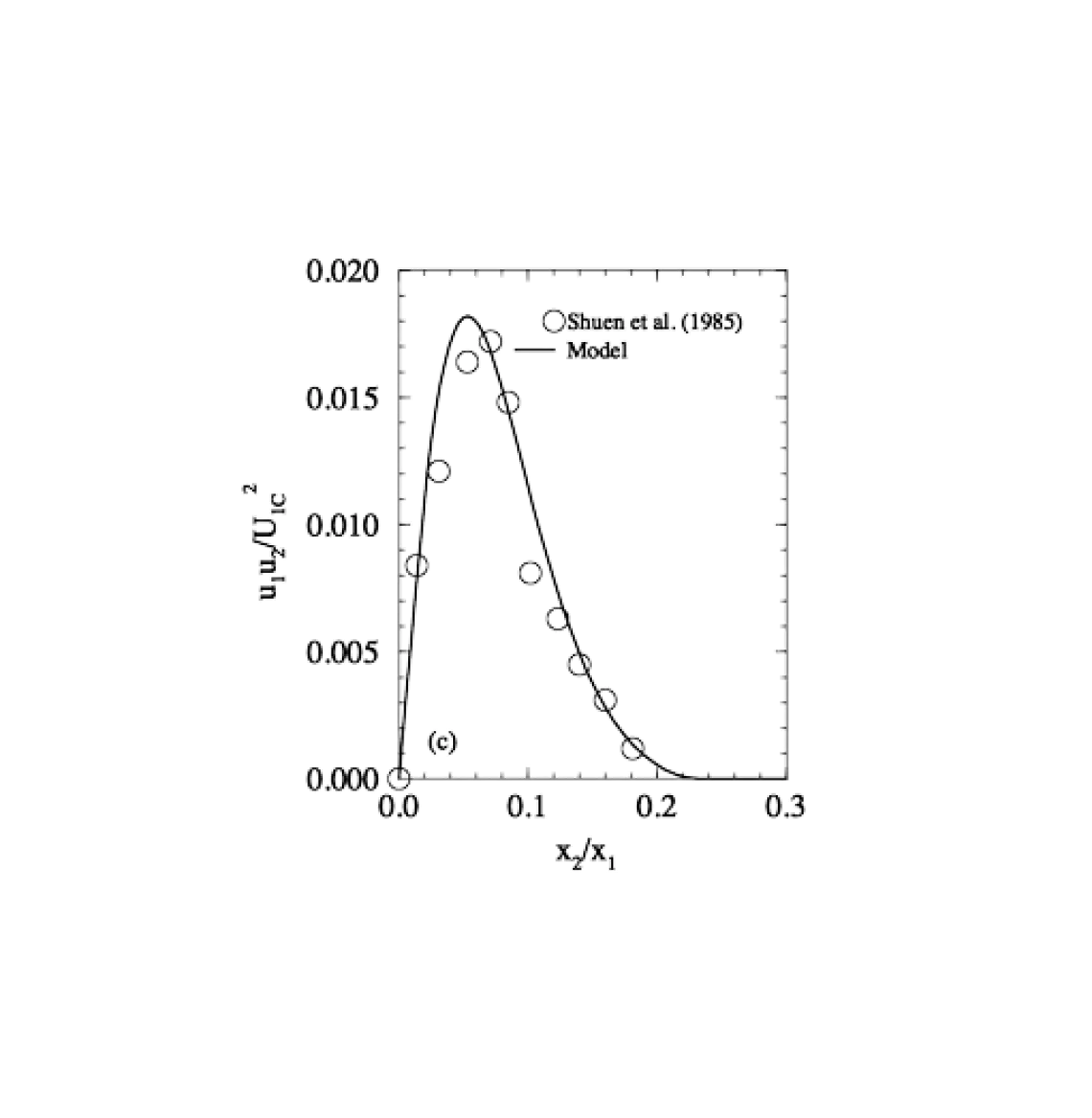
We have used the pdf approach extensively to derive 'fluid' models for a dispersed phase of particles or droplets in turbulent flows. First, a transport equation for the phase space density of the relevant variables is derived through the application of the Liouville's theorem. The ensemble average of this equation over a large number of realizations then yields a transport equation for the PDF of the droplet properties such as velocity and temperature. The process of ensemble averaging, however, results in the appearance of unclosed terms, manifesting the well-known closure problem in turbulence. We have applied a variety of closure schemes to this problem and developed fluid-like (continuum) transport equations for various moments of the dispersed phase. These transport equations take the forms of partial differential equations (PDE's) and can be integrated using standard methods.

Stochastic Modeling

We use the method of Time Series Analysis for our stochastic modeling. The method allows for incorporation of inherent turbulence features such as temporal and spatial correlations. Our stochastic models provide synthetic velocity fluctuations that can be added to mean velocities generated by RANS or any other means. The result is instantaneous velocity that closely mimics the properties of real turbulence in a statistical sense. Our stochastic models have been used in different codes, including Fourier spectral as well as ANSYS/Fluent to simulate particle dispersion. We also use our stochastic models to generate inlet turbulence fluctuation in DSEM.

Combustion Model

The main combustion model implemented in DSEM is Filtered Mass Density Function (FMDF). FMDF is based on a pdf transport equation that is solved with a Lagrangian method using Monte-Carlo particles. The implementation of FMDF in the DSEM code required new algorithms in order to preserve the desirable features of DSEM. These new algorithms extend the application of FMDF to unstructured grids and complex geometries. In the existing Finite Difference (FD) codes, an overlaid grid is typically used for calculation of the averages from the flow properties obtained on the Lagrangian particles. This approach proved not to be applicable to a topologically complex and unstructured grid. Furthermore, the particle search and tracking algorithms used in the FD codes are not directly extendable to an unstructured grid. We used the DSEM’s mapping method to develop new algorithms for particle search, tracking, and averaging in an efficient manner.

In addition to using FMDF, the DSEM code is also capable of simulating reacting flows without using a combustion model. This so-called "no-model" combustion simulation is stabilized using the explicit spectral filtering that is also used for ILES.

No-Model Combustion

The video depicts air passing over a cavity with a ramped forward facing wall. A small round injector is releasing fuel (Ethylene) inside the cavity. The top plot shows the fuel mass fraction, and the bottom plot is the product mass fraction (CO2). Both plots are colored by temperature.

Shock Capturing

For simulation of supersonic flows, a shock capturing method based on Entropy Viscosity (EV) method is implemented in the DSEM code. The EV method is modified to work with the discontinuous method and in unstructured grid. Since shocks generate significant entropy, a sensor based on entropy production can be used to detect shock regions in the flow. The entropy residual is also used to calculate the magnitude of the artificial viscosity needed to resolve the shock on the computational grid.

Summary

In summary, we truly specialize in computational fluid dynamics, particularly turbulent reacting flows. We have access to numerous closed source research-grade codes, commercial codes, and open source codes. We have experience implementing and using a wide range of models, each with their own strengths and shortcomings. There is no problem too small or too large, it all boils down to a question of computational time, which can be addressed with our wide variety of computing resources.